
Prof. Han's is an experimentalist on soft matter physics and is interested in statistical physics. He has published 56 first-author or corresponding-author papers, including 5 on Nature or Science. He received the Achievement in Asia Award by OCPA in 2014 and the 14th Chinese Young Scientist Award in China in 2016.
Currently his group studies crystalline and amorphous solids, their melting and solid-solid transitions using colloids as model systems. His team made the first observations of the following phenomena with the single-particle kinetics: crystal surface premelting, homogeneous 3D crystal melting, homogeneous solid-solid transition, grain boundary roughening, glass transition in non-spherical-particle system. Recently his team discovered of a novel type of surface wetting phenomenon as the precursor of crystal-crystal transition in analogous to premelting and made important contributions on novel topics such as: polycrystal-glass transition, glass surface melting, generalization of the Hall-Petch behaviors form polycrystals to crystal-amorphous composites. Prof. Han also studied topics in statistical physics, including phase-space networks of spin systems, configurational temperatures, and Brownian motion of non-spherical particles.
2007 - present, Assistant/Associate/Full Professor, Physics Department, Hong Kong University of Science and Technology
2004 - 2007, Postdoc, University of Pennsylvania, USA
2003, Ph.D. in Physics, University of Chicago, USA
1998, B.S. in Physics, Peking University, China
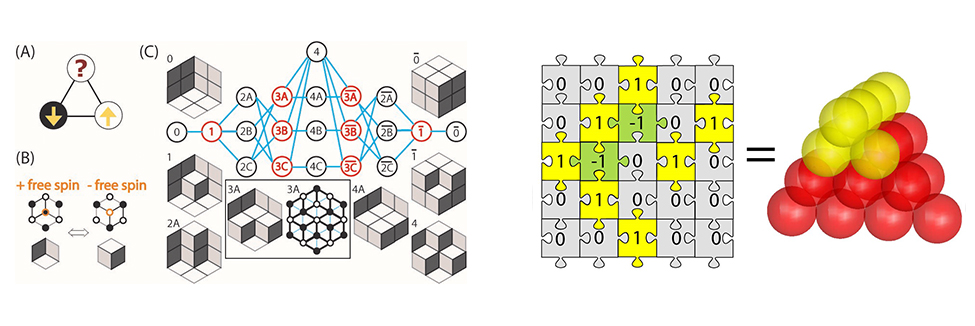
The phase space or state space of a system with discrete degrees of freedom can be viewed as a network: each node represents a state, and each edge represents a low-energy transition pathway between two states, e.g., a zero-energy mode. We map the exact ground states of various spin models (the antiferromagnet Ising spins on a triangular lattice, the six-vertex model (i.e., square ice), and ±J spin glasses) and phase spaces of 1D and 2D lattice gases to complex networks. Thus, the network analysis can be applied to phase-space studies. The phase spaces, in turn, establish a new class of complex networks (or random matrices) with unique topology characterized by the novel Gaussian spectral density. Although computer can only handle small systems as their phase spaces are huge, we mathematically prove that their spectral densities become exactly Gaussian when systems become infinitely large. The phase spaces of different models under different boundary conditions share some common features. The systems with long- and short-range correlations in real space exhibit fractal and non-fractal phase spaces, respectively, which supports the conjecture in Tsallis statistics. The connectivity distribution, community structures, and fractal structures change drastically at the ferromagnetic-to-glass phase transition. The network community analysis provides a way to describe and simplify the complex entropy landscape.
One by-product in the phase-space construction is a one-to-one mapping between the 2D six-vertex model and 3D sphere stackings. In contrast to cube stackings (i.e., antiferromagnet Ising spins on 2D triangular lattice) which has been intensively studied in combinatorics as plane partition, the sphere stacking has similar combinatorics problems but rarely been explored. We find that some sphere packing follows a mock theta function. We conjecture all models could be mapped to stackings in high dimensions.
References:
[1] Phase-space networks of geometrically frustrated systems, Yilong Han*, Phys. Rev. E 80, 051102 (2009)
[2] Phase-space networks of the six-vertex model under different boundary conditions, Yilong Han*, Phys. Rev. E 81, 041118 (2010)
[3] Self-similarity of phase-space networks of frustrated spin models and lattice gas models, Yi Peng, Feng Wang, Michael Wong, and Yilong Han*, Phys. Rev. E 84, 051105 (2011)
[4] Ground-state phase-space structures of two-dimensional ±J spin glasses: A network approach, Xin Cao, Feng Wang, and Yilong Han*, Phys. Rev. E 91, 062135 (2015)
[5] Geometric frustration in buckled colloidal monolayers, Y. Han*, Y. Shokef*, A. Alsayed, P. Yunker, T. C. Lubensky and A. G. Yodh, Nature 456, 898-903 (2008)